Chi Square Goodness of Fit Test Example Pdf
It is used to determine whether the distribution of cases eg participants in a single categorical variable eg gender consisting of two groups. The Chi-square goodness-of-fit test extends inference on proportions to more than two proportions by enabling us to determine if a particular population distribution has changed from a specified form.
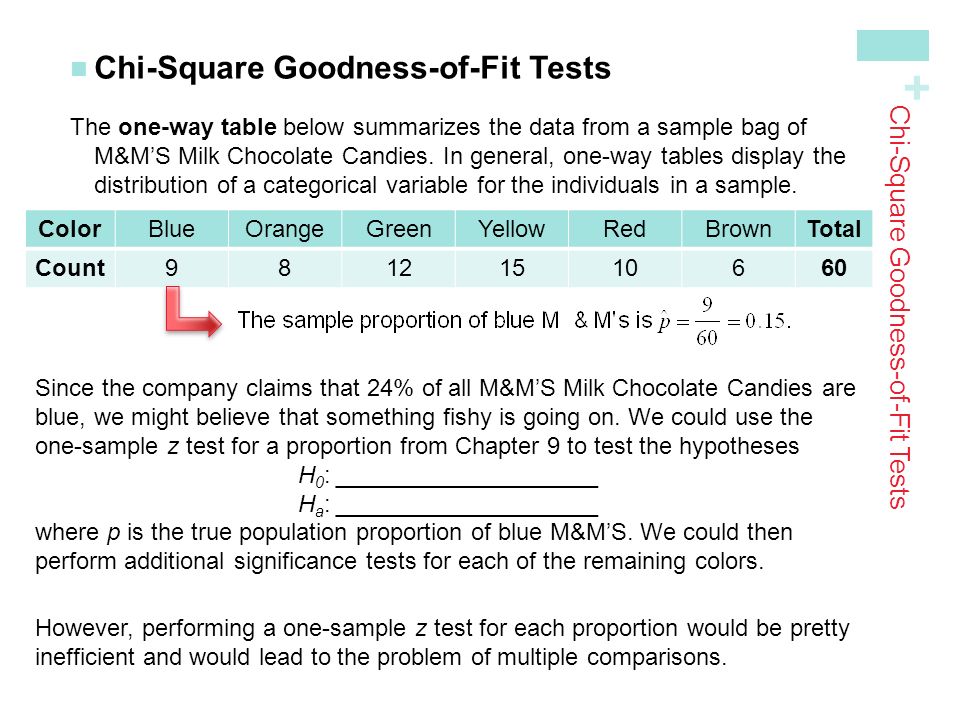
Section 11 1 Chi Square Goodness Of Fit Tests Ppt Download
Chi-Square Test for Goodness of Fit after Applied Statistics by HinkleWiersmaJurs Scientists will often use the Chi-square χ 2 test to determine the goodness of fit between theoretical and experimental data.
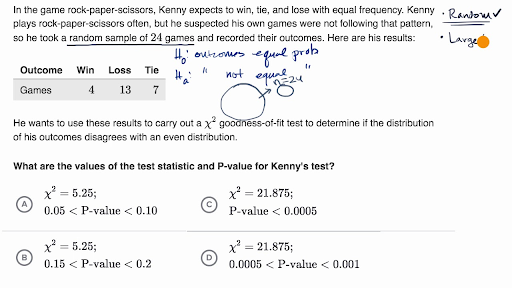
. Perform a chi-square goodness of fit test. Chi-Square goodness of fit test is a non-parametric test that is used to find out how the observed value of a given phenomena is significantly different from the expected value. In this test we compare observed values with theoretical or expected values.
Chi-Square Test for Goodness of Fit The chi-square goodness-of-fit test is a single-sample nonparametric test also referred to as the one-sample goodness-of-fit test or Pearsons chi-square goodness-of-fit test. Section 103 examines the chi square goodness of flt test and Section 104 presents a chi square test for independence of two variables. It answers the question of how well do experimental data fit expectations.
The Chi-square statistic takes the form of. For red we have 50 100 2 100 25. Using Excel we find CHIDIST036771 05443 This is not close to being statistically significant and we cannot.
Let us consider r boxes B1Br and throw n balls X1Xn into these boxes inde pendently of each other with probabilities PXi B1 p1 PXi Br pr so that p1. 22 2705 697 224 232 00918 02759 03677 697 232 χ To calculate the degrees of freedom observe that we have two groups ie k2 and that we did not need to estimate any population parameters ie m0. 2 Unless you have counts you cannot use χ methods _____ 2With χ the alternative hypothesis can be one-sided or two-sided.
To test this hypothesis he records the number of customers that come into the shop on a given week and finds the following. Df k-1 where k of categories in variable. Describe the chi-square test statistic.
A chi-square goodness of fit test revealed that the sample proportions collected did differ significantly from that of the population X 2 5 N 90. 1 Exercise8 Chi square test test for association and goodness of fit test for goodness of fit If 0 i i12n is a set of observed experimental frequencies and Ei i12n is the corresponding set of expected theoretical or hypothetical frequencies then. Goodness of Fit Mendel has no way of solving this problem.
Chi-squared goodness-of-fit test is based on a probabilistic result that we will prove in this section. Chi-Square Goodness-of-Fit Test Given that we observed 700 births under the null hypothesis the expected number of births on any day is 700 17 100 In this example the null hypot hesis exactly specifies that each p i17. In Chi-Square goodness of fit test the term goodness of fit is used to compare the observed sample distribution with the expected probability distribution.
1 2 r B1 B2. Karl Pearson and RA. In other words when you draw a random sample do the observed proportions follow the values that theory suggests.
Determine the test distribution to use Chi Square tests use X2 distribution. And draw a picture. For brown we have 42 100 2 100 3364.
For yellow we have 46 100 2 100 2916. Chi-Square Goodness of Fit test This is used when you have one independent variable and you want to compare an observed frequency-distribution to a theoretical expected frequency-distribution. Following our now standard notation we write.
Br p1 p2 pr Figure 101. Suppose a shop owner claims that an equal number of customers come into his shop each weekday. I Flinger M.
His winnings are directly proportional to the number of sixes recorded. In this case df 2-1 1. Pr 1.
For this example well use alpha 05 3. We start with a theory for how the offspring will be distributed. The chi-square test is a goodness of fit test.
Describe the chi-square distributions. Goodnessoffit tests use. Define the rejection regions.
The chi-square test can be used to estimate how closely the distribution of a categorical variable matches an expected distribution the goodness-of-fit test or to estimate whether two categorical variables are independent of one another the test of independence. A gambler plays a game that involves throwing 3 dice in a succession of trials. For example χ25 is the chi-squared curve withdf 5.
Value of Chi-Square goodness of fit test is calculated by using the formula X2 H Oi FEi2 Ei I. State the decision rule. The hypothesis tests we have looked at so far tests for one mean and tests for two means have compared a calculated test statistic to the standard normal distribution or the tdistribution.
Oi is the observed frequency count for ith level of the categorical variable and Ei is the expected frequency count for ith level of the categorical variable. The chi square test of independence is a natural extension. The term chi square pro-.
Hence the degrees of freedom is 210 1. CHI-SQUARE TEST OF GOODNESS OF FIT 1. These two sets of frequencies through a formal hypothesis test known as a chisquared χ2 goodnessoffit test.
Fisher developed the chi-square test for this purpose. We then total all of these contributions and determine that our chi-square statistic is 12544 2209 009 25 2916 3364 23542. Describe uses of the chi-square test.
Chi-Square Goodness of Fit Test. Chi-Square The test objective is to formalize the approach of matchin g a theoretical distribution across a histographic representation of the data The data ranggqye is divided into k intervals and the fre quency count O i for each interval is tabulated The expected frequency E i based on the theoretical pdf f with. Using Appendix 6 critical value df1 area in tail - 05 384 5.
Goodness-of-fit Test This is a test to see how well on sample proportions of categories match-up with the known population proportions. Similar to how a binomial random variable X takes on values x Further this terminology implies that we use a chi-squaredcurve to calculate probabilities for X2. For the alternative hypothesis there are 6 degrees of freedom 7 p i but they must sum to 1.
I will talk about a chi-squared random variable Such a random variable will be denoted by X2 and take on values χ2. 102 The Chi Square Distribution The chi square distribution is a theoretical or mathematical distribution which has wide applicability in statistical work. The chi-square goodness of fit test evaluates whether proportions of categorical or discrete outcomes in a sample follow a population distribution with hypothesized proportions.
Up to 24 cash back A A z-test of proportions B a two-sample test of means C a paired t-test D a chi-square test Why. Describe the cell counts required for the chi-square test. If the dice are fair what is the probability distribution that.
The basic practice of statistics 6th ed. Chi-Square goodness of fit test. Analysts frequently use the chi-square goodness of fit test to determine.
For the example described above there is a.

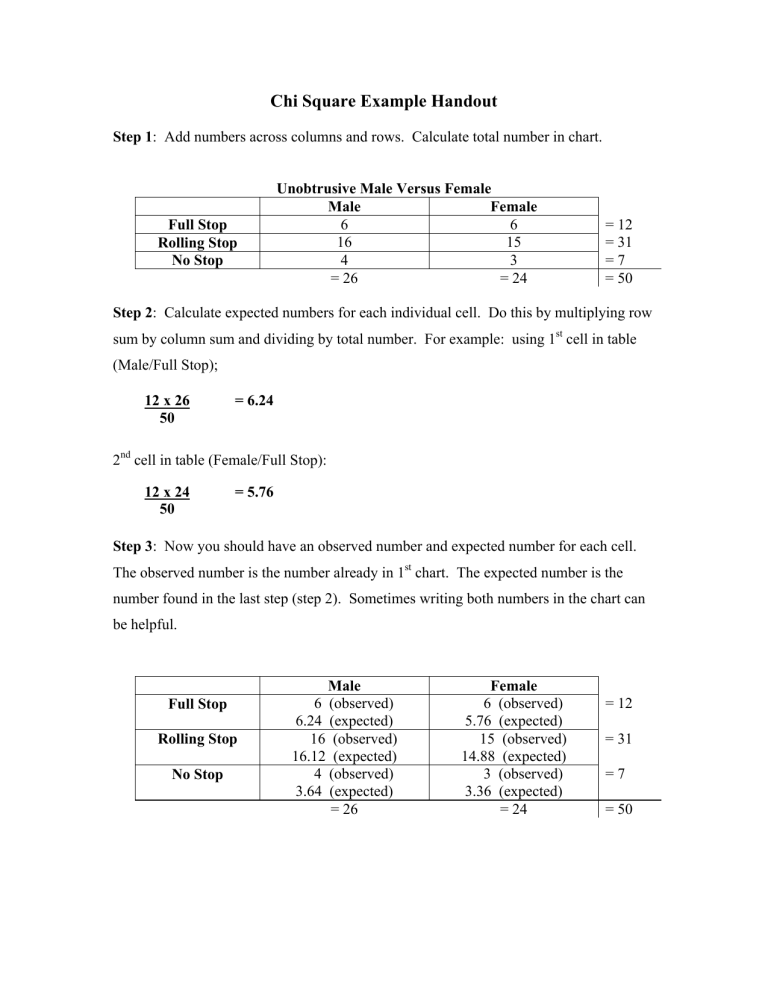
0 Response to "Chi Square Goodness of Fit Test Example Pdf"
Post a Comment